In the last lesson, you were introduced to decimal numbers. Decimal places change by a factor of 10. For example, let's look at the number 3,247.8956 below.
| 3 | x | 1000 | thousands |
| 2 | x | 100 | hundreds |
| 4 | x | 10 | tens |
| 7 | x | 1 | ones |
| 8 | x | 0.1 | tenths |
| 9 | x | 0.01 | hundredths |
| 5 | x | 0.001 | thousandths |
| 6 | x | 0.0001 | ten-thousandths |
A decimal number can have a whole-number part and a fractional part.
Here we will convert 6/26 so you can write it as a decimal. The 6 above the bar is called the numerator and the 26 below the bar is called the denominator. To convert 6/26 so you can write it as a decimal, simply divide the numerator by the denominator like this: = 6/26 = 6 ÷ 26 = 0.23076923 Therefore, the solution to 6/26 as a decimal is as. Write 26/45 as a decimal. The fraction 26/45 is equal to 0.7778 when converted to a decimal. See below detalis on how to convert the fraction 26/45 to a decimal value. Fraction to Decimal Converter. Enter a fraction value: Ex.: 1/2, 2 1/2, 5/3, etc. Note that 2 1/2 means two and half =.
| Mixed Number | - Expanded Form - | Decimal Form |
| = (5 x 10) + ( 7 x 1) | + (4 x ) + (9 x ) | = 57.49 |
| - whole-number part - | - fractional part - |
In this lesson, you will learn how to read and write decimals. You may use our Place Value and Decimals Chart (PDF) as a visual reference for the examples presented in this lesson.
Example 1: Write each mixed number as a decimal.
| Mixed Number | Decimal |
| 52.3000 | |
| 973.4100 | |
| 31.2670 | |
| 1,842.0056 |
Example 2: Write each phrase as a mixed number and as a decimal.
| phrase | mixed number | decimal |
| five and three tenths | 5.300000 | |
| forty-nine and one hundredth | 49.010000 | |
| two hundred sixteen and two hundred thirty-one thousandths | 216.231000 | |
| nine thousand, ten and three hundred fifty-nine ten-thousandths | 9,010.035900 | |
| seventy-six thousand, fifty-three and forty-seven hundred-thousandths | 76,053.000470 | |
| two hundred twenty-nine thousand and eighty-one millionths | 229,000.000081 |
Look at the mixed numbers in the examples above. You will notice that the denominator of the fractional part is a factor of 10, making it is easy to convert to a decimal. Let's look at some examples in which the denominator is not a factor of 10.
Example 3: Write each mixed number as a decimal.
Analysis: A fraction bar tells us to divide. In order to do this, we must convert or change the fractional part of each mixed number to decimal digits. We will do this by dividing the numerator of each fraction by its denominator.
|
Alternate Method: It should be noted that some of the fractions above could have been converted to decimals using equivalent fractions. For example:
Example 4: When asked to write two hundred thousandths as a decimal, three students gave three different answers as shown below. Which student had the correct answer?
Student 1: 200,000.
Student 2: 0.200
Invisor 3 13 download free. Student 3: 0.00002
Analysis: Let's use our place value chart to help us analyze this problem.
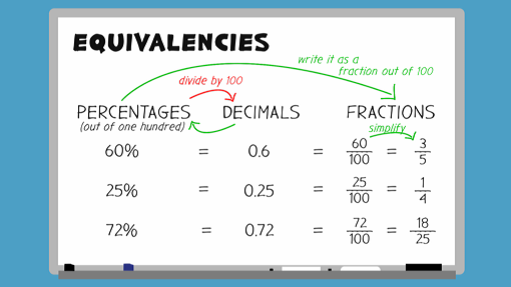
Write 26/20 As A Decimal
|
Let's look at the expanded form of each decimal to help us find the correct answer.
|
Answer: Thus, two hundred thousandths is 0.200, so Student 2 had the correct answer.
As you can see, decimals are named by the place of the last digit. Notice that in Example 4, the answer given by Student 3 was two hundred-thousandths. This phrase has a hyphen in it. The hyphen is an important piece of information that helps us read and write decimals. Let's look at some more examples.
Example 5: Write each phrase as a decimal.
| phrase | analysis | fraction | decimal |
| three hundred ten thousandths | 310 thousandths | 0.310 | |
| three hundred ten-thousandths | 300 ten-thousandths | 0.0300 |
Example 6: Write each phrase as a decimal.
| phrase | analysis | fraction | decimal |
| eight hundred thousandths | 800 thousandths | 0.800 | |
| eight hundred-thousandths | 8 hundred-thousandths | 0.00008 |
Example 7: Write each phrase as a decimal.
| phrase | analysis | fraction | decimal |
| seven hundred millionths | 700 millionths | 0.000700 | |
| seven hundred-millionths | 7 hundred-millionths | 0.00000007 |
In Examples 5 through 7, we were asked to write phrases as decimals. Some of the words in the phrase indicate the place-value positions, and other words in the phrase indicate the digits to be used. Now let's look at some examples in which we write these kinds of decimals using words.
Example 8: Write each decimal using words. Free live recording software.
| decimal | analysis | phrase |
| 0.110 | 110 thousandths | one hundred ten thousandths |
| 0.0100 | 100 ten-thousandths | one hundred ten-thousandths |
Example 9: Write each decimal using words.
| decimal | analysis | phrase |
| 0.400 | 400 thousandths | four hundred thousandths |
| 0.00004 | 4 hundred-thousandths | four hundred-thousandths |
| Example 10: | Write the following decimal using words. Roll your mouse over each digit for help. |
| Answer: | The decimal 1,729,405.008365 is written as: |
one million, seven hundred twenty-nine thousand, four hundred five and eight thousand, three hundred sixty-five millionths

Write 26/20 As A Decimal
|
Let's look at the expanded form of each decimal to help us find the correct answer.
|
Answer: Thus, two hundred thousandths is 0.200, so Student 2 had the correct answer.
As you can see, decimals are named by the place of the last digit. Notice that in Example 4, the answer given by Student 3 was two hundred-thousandths. This phrase has a hyphen in it. The hyphen is an important piece of information that helps us read and write decimals. Let's look at some more examples.
Example 5: Write each phrase as a decimal.
| phrase | analysis | fraction | decimal |
| three hundred ten thousandths | 310 thousandths | 0.310 | |
| three hundred ten-thousandths | 300 ten-thousandths | 0.0300 |
Example 6: Write each phrase as a decimal.
| phrase | analysis | fraction | decimal |
| eight hundred thousandths | 800 thousandths | 0.800 | |
| eight hundred-thousandths | 8 hundred-thousandths | 0.00008 |
Example 7: Write each phrase as a decimal.
| phrase | analysis | fraction | decimal |
| seven hundred millionths | 700 millionths | 0.000700 | |
| seven hundred-millionths | 7 hundred-millionths | 0.00000007 |
In Examples 5 through 7, we were asked to write phrases as decimals. Some of the words in the phrase indicate the place-value positions, and other words in the phrase indicate the digits to be used. Now let's look at some examples in which we write these kinds of decimals using words.
Example 8: Write each decimal using words. Free live recording software.
| decimal | analysis | phrase |
| 0.110 | 110 thousandths | one hundred ten thousandths |
| 0.0100 | 100 ten-thousandths | one hundred ten-thousandths |
Example 9: Write each decimal using words.
| decimal | analysis | phrase |
| 0.400 | 400 thousandths | four hundred thousandths |
| 0.00004 | 4 hundred-thousandths | four hundred-thousandths |
| Example 10: | Write the following decimal using words. Roll your mouse over each digit for help. |
| Answer: | The decimal 1,729,405.008365 is written as: |
one million, seven hundred twenty-nine thousand, four hundred five and eight thousand, three hundred sixty-five millionths
Summary: You learned how to read and write decimals in this lesson. When writing a mixed number as a decimal, the fractional part must be converted to decimal digits. Decimals are named by the place of the last digit. The hyphen is an important indicator when reading and writing decimals. When writing a phrase as a decimal, some of the words indicate the place-value positions, and other words indicate the digits to be used.
Exercises
In Exercises 1 and 2, click once in an ANSWER BOX and type in your answer; then click ENTER. After you click ENTER, a message will appear in the RESULTS BOX to indicate whether your answer is correct or incorrect. To start over, click CLEAR.
| In Exercises 3 through 5, read each question below. Select your answer by clicking on its button. Feedback to your answer is provided in the RESULTS BOX. If you make a mistake, choose a different button. |
| 3. | Which of the following is equal to seven hundred five thousand and eighty-nine ten-thousandths? |
| 4. | Which of the following is equal to 9,842.1039? |
| 5. | Which of the following is equal to five hundred-thousandths? |
The fraction 26/9 is equal to 2.8888888888889 when converted to a decimal. See below detalis on how to convert the fraction 26/9 to a decimal value.
How to convert from fraction to decimal?
To easily convert a fraction to a decimal, divide the numerator (top number) by the denominator (bottom number).Example 1: How to convert 4/8 to a decimal?
- Step 1:
Divide 4 by 8: 4 ÷ 8 = 1 ÷ 2 = 0.5 How to get apple music on xbox.
- Step 2:
Multiply the result by 100 and add the decimal sign: 0.5 × 100%
- Answer: 4/8 = 50%
Example 2: How to convert 1 1/3 to a decimal?
Write 26/10 As A Decimal
- Step 1:
Divide 1 by 3: 1 ÷ 3 = 0.3333
- Step 2:
Add this value to the the integer part: 1 + 0.3333 = 1.3333
- Step 3:
Multiply the result by 100 and add the decimal sign: 1.3333 × 100%
- Answer: 1 1/3 = 133.33%
Write 7 8 As A Decimal
Note: the result was rounded to 2 decimal places.
Fractions of inches to decimal inches and millimeters (mm)
| fraction | inches | mm |
|---|---|---|
| 1/64 | 0.0156 | 0.3969 |
| 1/32 | 0.0313 | 0.7938 |
| 3/64 | 0.0469 | 1.1906 |
| 1/16 | 0.0625 | 1.5875 |
| 5/64 | 0.0781 | 1.9844 |
| 3/32 | 0.0938 | 2.3813 |
| 7/64 | 0.1094 | 2.7781 |
| 1/8 | 0.1250 | 3.1750 |
| 9/64 | 0.1406 | 3.5719 |
| 5/32 | 0.1563 | 3.9688 |
| 11/64 | 0.1719 | 4.3656 |
| 3/16 | 0.1875 | 4.7625 |
| 13/64 | 0.2031 | 5.1594 |
| 7/32 | 0.2188 | 5.5563 |
| 15/64 | 0.2344 | 5.9531 |
| 1/4 | 0.2500 | 6.3500 |
| fraction | inches | mm |
|---|---|---|
| 17/64 | 0.2656 | 6.7469 |
| 9/32 | 0.2813 | 7.1438 |
| 19/64 | 0.2969 | 7.5406 |
| 5/16 | 0.3125 | 7.9375 |
| 21/64 | 0.3281 | 8.3344 |
| 11/32 | 0.3438 | 8.7313 |
| 23/64 | 0.3594 | 9.1281 |
| 3/8 | 0.3750 | 9.5250 |
| 25/64 | 0.3906 | 9.9219 |
| 13/32 | 0.4063 | 10.3188 |
| 27/64 | 0.4219 | 10.7156 |
| 7/16 | 0.4375 | 11.1125 |
| 29/64 | 0.4531 | 11.5094 |
| 15/32 | 0.4688 | 11.9063 |
| 31/64 | 0.4844 | 12.3031 |
| 1/2 | 0.5000 | 12.7000 |
| fraction | inches | mm |
|---|---|---|
| 33/64 | 0.5156 | 13.0969 |
| 17/32 | 0.5313 | 13.4938 |
| 35/64 | 0.5469 | 13.8906 |
| 9/16 | 0.5625 | 14.2875 |
| 37/64 | 0.5781 | 14.6844 |
| 19/32 | 0.5938 | 15.0813 |
| 39/64 | 0.6094 | 15.4781 |
| 5/8 | 0.6250 | 15.8750 |
| 41/64 | 0.6406 | 16.2719 |
| 21/32 | 0.6563 | 16.6688 |
| 43/64 | 0.6719 | 17.0656 |
| 11/16 | 0.6875 | 17.4625 |
| 45/64 | 0.7031 | 17.8594 |
| 23/32 | 0.7188 | 18.2563 |
| 47/64 | 0.7344 | 18.6531 |
| 3/4 | 0.7500 | 19.0500 |
| fraction | inches | mm |
|---|---|---|
| 49/64 | 0.7656 | 19.4469 |
| 25/32 | 0.7813 | 19.8438 |
| 51/64 | 0.7969 | 20.2406 |
| 13/16 | 0.8125 | 20.6375 |
| 53/64 | 0.8281 | 21.0344 |
| 27/32 | 0.8438 | 21.4313 |
| 55/64 | 0.8594 | 21.8281 |
| 7/8 | 0.8750 | 22.2250 |
| 57/64 | 0.8906 | 22.6219 |
| 29/32 | 0.9063 | 23.0188 |
| 59/64 | 0.9219 | 23.4156 |
| 15/16 | 0.9375 | 23.8125 |
| 61/64 | 0.9531 | 24.2094 |
| 31/32 | 0.9688 | 24.6063 |
| 63/64 | 0.9844 | 25.0031 |
| 1 | 1.0000 | 25.4000 |
